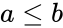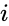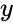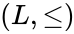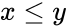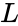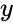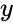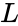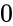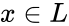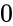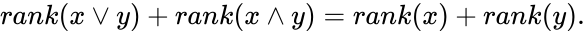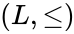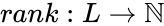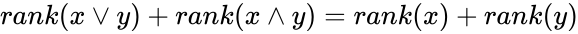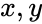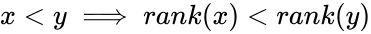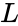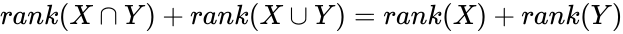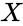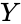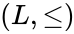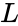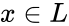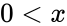A lattice is said to be modular if the following identity holds  whenever
whenever 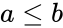 . Modular lattices include the following:
. Modular lattices include the following:
- Distributive lattices
- The lattice of normal subgroups of a group.
- The lattice of subgroups of abelian group.
- The lattice of subspaces of a vector space.
- More generally, the lattice of submodules of a module over a ring.
- The lattice of ideals in a ring.
Modularity is essentially the condition needed for some version of the Jordan-Hölder theorem to hold. Recall that a composition sequence in a poset is a sequence  such that for each
such that for each 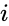 , there is no
, there is no 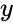 such that
such that  . Then one has the following result:
. Then one has the following result:
Let 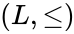 be a modular lattice. Suppose
be a modular lattice. Suppose 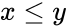 are elements of
are elements of 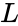 . If there is a composition sequence starting with
. If there is a composition sequence starting with  and ending with
and ending with 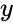 , then all such composition sequences have the same length. If this length is
, then all such composition sequences have the same length. If this length is  , then any chain from
, then any chain from  to
to 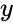 has length at most
has length at most  .
.
Suppose that 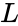 is a modular lattice with a least element
is a modular lattice with a least element 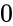 , and suppose that for every
, and suppose that for every 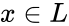 , there is a composition sequence from
, there is a composition sequence from 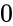 to
to  . Then one can define the "rank" of
. Then one can define the "rank" of  to be the length of such a composition sequence, and the following identity holds:
to be the length of such a composition sequence, and the following identity holds: 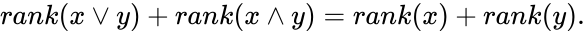 These assumptions hold in particular for finite modular lattices.
These assumptions hold in particular for finite modular lattices.
Conversely, if 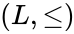 is a finite lattice with a function
is a finite lattice with a function 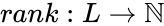 such that
such that 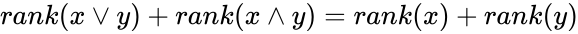 holds for every
holds for every 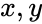 , and such that
, and such that 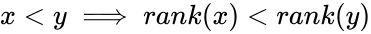 , then
, then 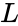 is modular.
is modular.
Modularity mainly appears in model theory through the notion of a modular pregeometry. A pregeometry is modular if the lattice of closed sets is modular. An equivalent condition is that the lattice of finite rank closed sets is modular, which is in turn equivalent to the identity 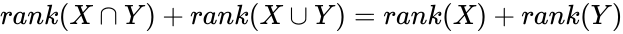 whenever
whenever 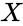 and
and 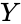 are closed sets (of finite rank). Modular pregeometries are essentially equivalent to projective planes and higher-dimensional generalizations (projective spaces).
are closed sets (of finite rank). Modular pregeometries are essentially equivalent to projective planes and higher-dimensional generalizations (projective spaces).
Conversely, if 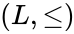 is a modular lattice with least element 0, there is a natural pregeometry structure on the atoms of
is a modular lattice with least element 0, there is a natural pregeometry structure on the atoms of 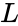 , i.e., the set of elements
, i.e., the set of elements 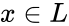 such that
such that 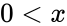 is a composition sequence.
is a composition sequence.
A (finite?) modular lattice is said to be atomic if every element is the join of the atoms below it. Atomic (finite?) modular lattices are essentially equivalent to modular pregeometries.
Modularity plays an important role in the (false) Zilber trichotomy conjecture, as well as results like the Buechler dichotomy and Zilber's theorem on countably categorical strongly minimal sets. ::: ::: :::
 whenever
whenever 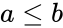 . Modular lattices include the following:
. Modular lattices include the following: